Interferometry

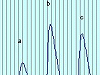
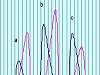
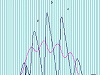
In Fort Collins, CO, SARA member Rodney Howe is doing hydrogen-line interferometry with a two-dish array and a Spectra-Cyber receiver. He sends us these images (clicking on each thumbnail will download the full high-resolution image):

 


Rodney Howe's 1420 MHz interferometer

This array consists of two eight-foot diameter parabolic TVRO dishes, fed with cylindrical waveguide feedhorns and equipped with Radio Astronomy Supplies low-noise amplifiers. The system uses the pseudo-polar mounts that came with the satellite dishes. The antennas are positioned along an east - west baseline adjustable from 20 to 22 wavelenghts, approximately 4.2 meters from feedhorn to feedhorn. All astonomy observations will be drift scans, as the dishes require manual allignment.

 thumbnail 1

 

thumbnail 5 The two-dish interferometer under construction. Howe emphasizes that mounting the dishes required the help of several strong teenagers. thumbnail 6

 

First fringes of the Sun, taken with the above interferometer

Samples are collected at 10 second intervals. There are 3 large peaks (a,b,c), these can be used to calculate the angular distance between the peaks comparing what it should look like mathematically to what has been recorded while the sun drifts through the beam of the antenna. Using the following formulae from Bill Lonc, Radio Astronomy Projects, 1996, we can substitute dimensions of the interferomter:

Sun drift scan angle in minutes = (angle * 4). Where 4 minutes = sideral
time. The angle: angle = (lambda / Distance * Cos (Declination)) *
(Pi/180)

where:

  • lambda = 21cm
  • Distance = 420cm (20*lambda)
  • Declination = +1.71 degrees (sun angle, the dishes are at 27 degrees elevation)
  • Pi radians = (Pi/180) = 57.29 degrees

So according to formula the minute spacing between the peaks in the
interferogram should = 11.47 minutes. (Minutes = (Angle * 4 = (21cm /
420cm * .9992 ) * 57.3)).

How does the recorded data look by comparison? The number of minutes
between the peaks in these data: from peak a to peak b = 11 minutes, from
peak b to peak c = 13 minutes, the average = 12 minutes. How close is this
to the theoretical? 11.47 minutes / 12 minutes = 95%

 thumbnail 2

 

Polarization studiy with the feedhorn elements at 30 degrees

It is interesting to adjust the feed element of both of the dishes (rotate the feedhorns so the brass element inside the 'can' is 0 degrees from the north/south position) and to compare the polarization interferogram with the original. The original interferogram had the feed elements positioned at a 30 degree angle from true north/south. Compare this with the feed elements directly facing north/south or at 0 degrees.

 thumbnail 3

 

Polarization studies with feedhorns orthogonal to each other

One feedhorn element is at 45 degrees from 0 (north/south), the other feedhorn element on the other dish is at 135 degrees from 0. Compare this with the original interferogram where both feedhorn elements are at 30 degrees from 0. The fringes begin to dissappear, and get closer to what you would see with just one dish, a continum scan.

 thumbnail 4